LINES & ANGLES

Parallel Lines, and Pairs of Angles

Parallel Lines

LINES are parallel if they are always the same distance apart (called "equidistant"), and will never meet. Just remember:

Always the same distance apart and never touching.

The red line is parallel to the blue line in both these cases:
Parallel Example 1Parallel Example 2
Example 1
Example 2

Parallel lines also point in the same direction.


Pairs of Angles

When parallel lines get crossed by another line (which is called aTRANSVERSAL), you can see that many angles are the same, as in this example:
These angles can be made into pairs of angles which have special names.


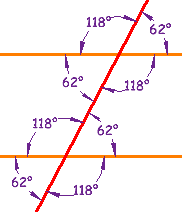Testing for Parallel Lines

Some of those special pairs of angles can be used to test if lines really are parallel: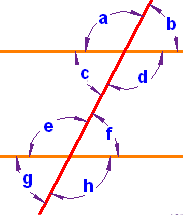 

If Any Pair Of ...Example:
CORRESPONDING ANGLES are equal, ora = e
ALTERNATE INTERIORE ANGLES are equal, orc = f
ALTERNATE EXTERIOR ANGLES are equal, orb = g
CONSECUTIVE INTERIOR ANGLES add up to 180°d + f = 180°
... then the lines are Parallel

Examples

These lines are parallel, because a pair ofCORRESPONDING ANGLES are equal.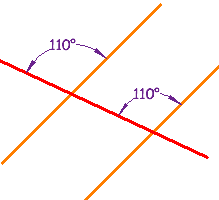
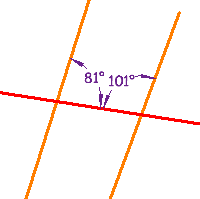These lines are not parallel, because a pair of CONSECUTIVE INTERIOR ANGLES do not add up to 180° (81° + 101° =182°)
These lines are parallel, because a pair of ALTERNATE INTERIORE ANGLES  are equal
S-BATCH