Rational Numbers
A Rational Number is a real number that can be written as a simple fraction (i.e. as a ratio).
Example:
1.5 is a rational number because 1.5 = 3/2 (it can be written as a fraction)
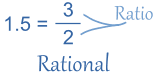
Here are some more examples:
| Number | As a Fraction | Rational? |
|---|---|---|
| 5 | 5/1 | Yes |
| 1.75 | 7/4 | Yes |
| .001 | 1/1000 | Yes |
| 0.111... | 1/9 | Yes |
| √2 (square root of 2) | ? | NO ! |
Oops! The square root of 2 cannot be written as a simple fraction! And there are many more such numbers, and because they are not rational they are called Irrational.
Another famous irrational number is Pi (π):
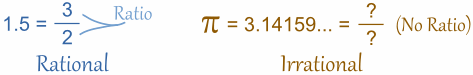
Formal Definition of Rational Number
More formally we would say:
A rational number is a number that can be in the form p/q
where p and q are integers and q is not equal to zero.
where p and q are integers and q is not equal to zero.
So, a rational number can be:
| p |
| q |
Where q is not zero
Examples:
| p | q | p / q | = |
|---|---|---|---|
| 1 | 1 | 1/1 | 1 |
| 1 | 2 | 1/2 | 0.5 |
| 55 | 100 | 55/100 | 0.55 |
| 1 | 1000 | 1/1000 | 0.001 |
| 253 | 10 | 253/10 | 25.3 |
| 7 | 0 | 7/0 | No! "q" can't be zero! |