Proportions
Proportion says that two ratios (or fractions) are equal.
Example:
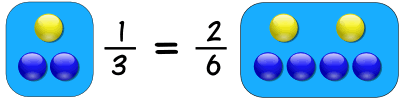
So 1-out-of-3 is equal to 2-out-of-6
The ratios are the same, so they are in proportion.
When things are "in proportion" then their relative sizes are the same.
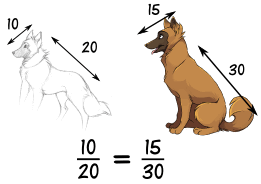
Here you can see that the ratios of head length to body length are the same in both drawings.
So they are proportional.
Making the head too long or short would look bad!
|  |
Working With Proportions
NOW, how do we use this?
Example: you want to draw the dog's head, and would like to know how long it should be:
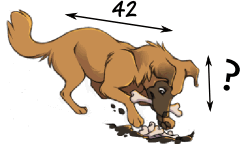
Let us write the proportion with the help of the 10/20 ratio from above:
| ? | = | 10 |
| 42 | 20 |
Now we solve it using a special method:

Multiply across the known corners,
then divide by the third number
then divide by the third number
And you get this:
? = (42 × 10) / 20 = 420 / 20 = 21
So you should draw the head 21 long.
Using Proportions to Solve Percents
A percent is actually a ratio! Saying "25%" is actually saying "25 per 100":
| 25% = | 25 |
| 100 |
We can use proportions to solve questions involving percents.
First, put what you know into this form:
| Part | = | Percent |
| Whole | 100 |
Example: what is 25% of 160 ?
The percent is 25, the whole is 160, and we want to find the "part":
| Part | = | 25 |
| 160 | 100 |
Find the Part:
Example: what is 25% of 160 (continued) ?
| Part | = | 25 |
| 160 | 100 |
Multiply across the known corners, then divide by the third number:

Part = (160 × 25) / 100 = 4000 / 100 = 40
Answer: 25% of 160 is 40.
Note: you could have also solved this by doing the divide first, like this:
Part = 160 × (25 / 100) = 160 × 0.25 = 40
Either method works fine.
We can also find a Percent:
Example: what is $12 as a percent of $80 ?
Fill in what you know:
| $12 | = | Percent |
| $80 | 100 |
Multiply across the known corners, then divide by the third number. This time the known corners are top left and bottom right:

Percent = ($12 × 100) / $80 = 1200 / 80 = 15%
Answer: $12 is 15% of $80
Or find the Whole:
Example: The sale price of a phone was $150, which was only 80% of normal price. What was the normal price?
Fill in what you know:
| $150 | = | 80 |
| Whole | 100 |
Multiply across the known corners, then divide by the third number:

Whole = ($150 × 100) / 80 = 15000 / 80 = 187.50
Answer: the phone's normal price was $187.50
Using Proportions to Solve Triangles
You can use proportions to solve similar triangles.
Example: How tall is the Tree?
Sam tried using a ladder, tape measure, ropes and various other things, but still couldn't work out how tall the tree was.
But then Sam has a clever idea ... similar triangles!
Sam measures a stick and its shadow (in meters), and also the shadow of the tree, and this is what he gets:
|  |
Now Sam makes a sketch of the triangles, and writes down the "Height to Length" ratio for both triangles:
| 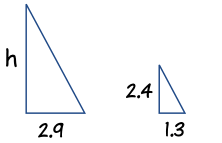 |
Multiply across the known corners, then divide by the third number:
h = (2.9 × 2.4) / 1.3 = 6.96 / 1.3 = 5.4 m (to nearest 0.1)
Answer: the tree is 5.4 m tall.
And he didn't even need a ladder!
The "Height" could have been at the bottom, so long as it was on the bottom for BOTH ratios, like this:
Let us try the ratio of "Shadow Length to Height":
|  |
Multiply across the known corners, then divide by the third number:
h = (2.9 × 2.4) / 1.3 = 6.96 / 1.3 = 5.4 m (to nearest 0.1)
It is the same calculation as before.
A "Concrete" Example
Ratios can have more than two numbers!
For example concrete is made by mixing cement, sand, stones and water.
 |
A typical mix of cement, sand and stones is written as a ratio, such as 1:2:6.
You can multiply all values by the same amount and you will still have the same ratio.
10:20:60 is the same as 1:2:6
So if you used 10 buckets of cement, you should use 20 of sand and 60 of stones.
|
Example: if you have just put 12 buckets of stones into a wheelbarrow, how much cement and how much sand should you add to make a 1:2:6 mix?
Let us lay it out in a table to make it clearer:
| Cement | Sand | Stones | |
|---|---|---|---|
| Ratio Needed: | 1 | 2 | 6 |
| You Have: | ? | ? | 12 |
You can see that you have 12 buckets of stones but the ratio says 6.
That is OK, you simply have twice as many stones as the number in the ratio ... so you need twice as much of everything to keep the ratio.
Here is the solution:
| Cement | Sand | Stones | |
|---|---|---|---|
| Ratio Needed: | 1 | 2 | 6 |
| You Have: | 2 | 4 | 12 |
And the ratio 2:4:12 is the same as 1:2:6 (because they show the same relative sizes)
Why are they the same ratio? In the 1:2:6 ratio there is 3 times more Stones as Sand (6 vs 2), and in the 2:4:12 ratio there is also 3 times more Stones as Sand (12 vs 4) ... similarly there is twice as much Sand as Cement in both ratios.
That is the good thing about ratios. You can make the amounts bigger or smaller and so long as the relative sizes are the same then the ratio is the same.
So the answer is: add 2 buckets of Cement and 4 buckets of Sand. (You will also need water and a lot of stirring....)
CLICK
CLICK